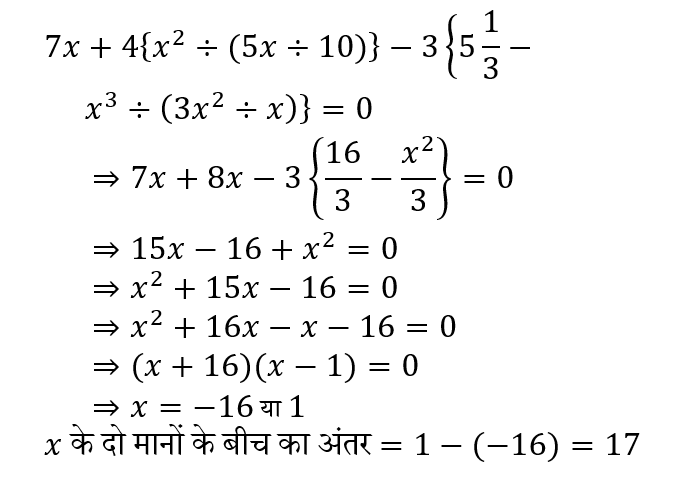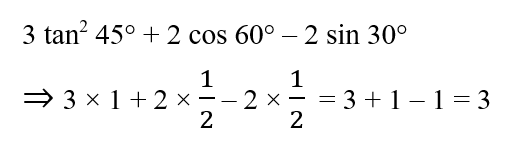Question 1:
(320 + 342 + 530 + 915) ÷ (20 + 22 – x + 18) = 43, then what is the value of x?
(320 + 342 + 530 + 915) ÷ (20 + 22 – x + 18) = 43 है, तो x का मान क्या है ?
Question 2: 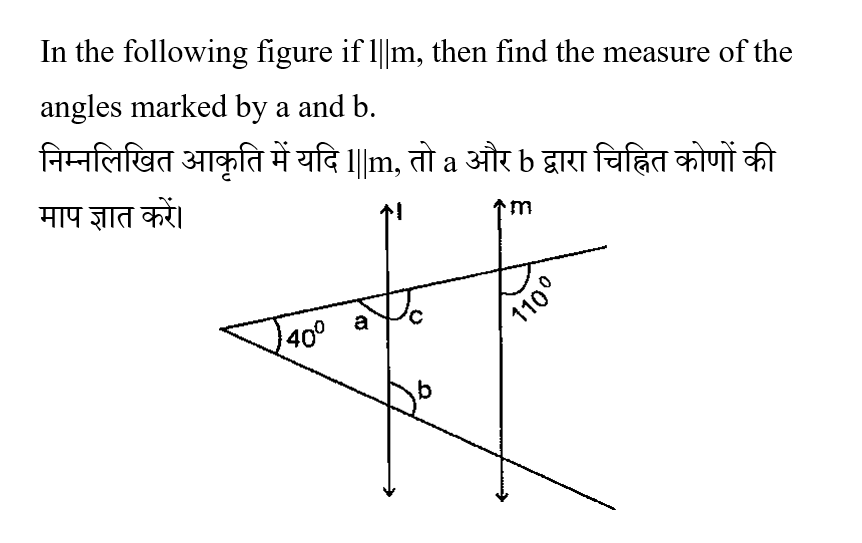
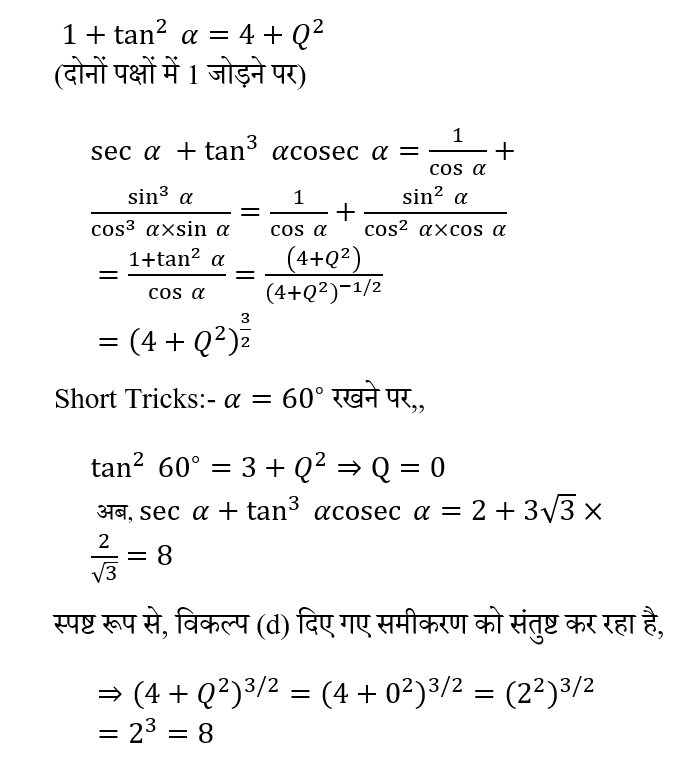
Question 3:
If the 8-digit number 888x53y4 is divisible by 72, then what will be the value of (7x + 2y) for the maximum value of y?
यदि 8- अंकीय संख्या 888x53y4, 72 से विभाज्य है, तो y के अधिकतम मान के लिए (7x + 2y) का मान क्या होगा?
Question 4: 
Question 5:
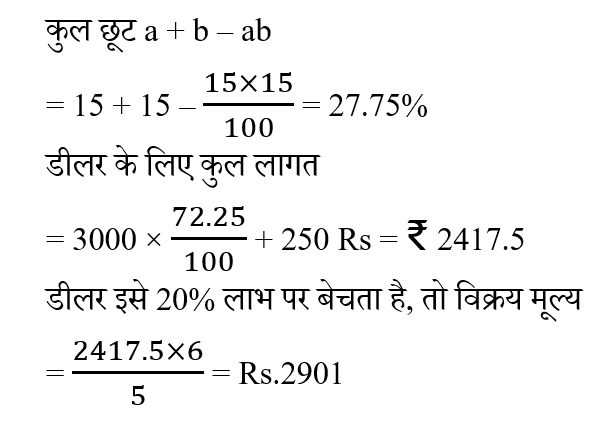
A dealer buys an item marked at ₹3,000 and gets successive discounts of 15% and 15%. He spends ₹250 on transportation and sells it at a profit of 20%. Find the selling price of the item.
एक डीलर ₹3,000 अंकित मूल्य की एक वस्तु खरीदता है और 15% एवं 15% की क्रमागत छूटें प्राप्त करता है। वह परिवहन पर ₹250 खर्च करता है और इसे 20% के लाभ पर बेचता है। वस्तु का विक्रय मूल्य ज्ञात करें।
Question 6: 
Question 7:
A person has some coins of denominations ₹10, ₹5 and ₹2. The ratio of the product of the number of ₹10 and ₹5 coins, the product of the number of ₹5 and ₹2 coins and the product of the number of ₹2 and ₹10 coins is 3:4:2 respectively. What is the minimum amount of money that person can have?
एक व्यक्ति के पास ₹10, ₹5 और ₹2 मूल्यवर्ग के कुछ सिक्के है। ₹10 और ₹5 के सिक्कों की संख्या के गुणनफल, ₹5 और ₹2 के सिक्कों की संख्या के गुणनफल और ₹2 और ₹ 10 के सिक्कों की संख्या के गुणनफल का अनुपात क्रमशः 3:4:2 है। उस व्यक्ति के पास न्यूनतम कितनी धनराशि हो सकती है ?
Question 8: 
Question 9: 
Question 10:
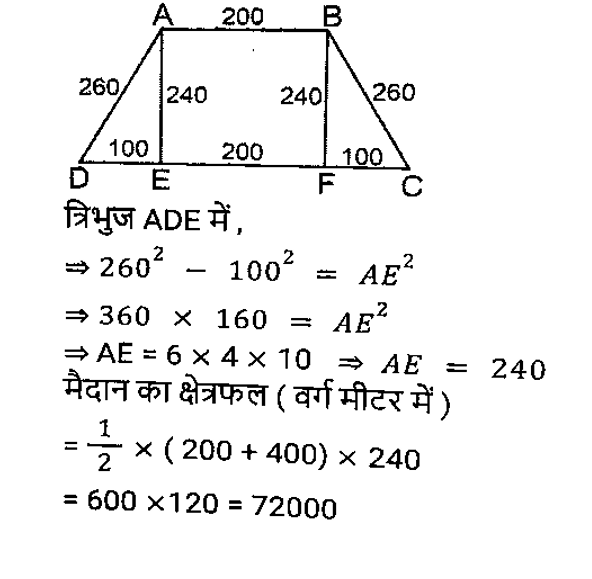
A field is in the shape of a trapezoid whose parallel sides are 200 m and 400 m long, while each of the other two sides is 260 m long. Find the area of this field (in square metres).
एक मैदान समलम्ब चतुर्भुज के आकार का है जिसकी समानांतर भुजाएं 200 मी और 400 मी लंबी हैं, जबकि अन्य दोनों में से प्रत्येक भुजा 260 मीटर लंबी है । इस मैदान का क्षेत्रफल (वर्ग मीटर में) ज्ञात करें ।